Molecular biologist, the late Francis H.C. Crick summarized one of our greatest intellectual challenges: to explain how consciousness¹ (1 Consciousness means a process of preconscious experienceability (subjectivity) leading to conscious experience (self-awareness).) can arise from below brain chemistry (Crick:1994b):

“We all know that quantum mechanics is the basis of chemistry, so no one can say that quantum mechanics is not important. But what people are trying to say is that there is something more than chemistry involved. And I can’t see that they have any grounds for that yet. Because they have not shown even in outline, not even as a sketch, what mysteries of the brain’s function would be explained if something of this type did take place.”

Stuart Hameroff and Sir Roger Penrose (Hameroff:1996a, Hameroff:1996b) took the challenge and developed the “Orch OR” theory of consciousness. Although their avant-garde model cannot be criticized on the basis that consciousness needs to bridge the gap between micro- and macro-events in the brain, it can be criticized for not being a proper biological model (Georgiev:2007). The philosophical basis of the model is neutral monism whereupon conscious episodes arise not from matter, but from a sequence of quantum state reductions (wave-function collapses). Superposition is separation of space-time curvature which self-collapses causing consciousness to connect to the structure of the universe. Therefore, the “Orch OR” theory connects the preconscious intrinsically to the fine-scale structure of the universe by orchestrated quantum-state reduction in space-time geometry (see (Hameroff:2017) for a review). How experiential and physical entities arise from this aspectless neutral entity as advanced by the “Orch OR” theory remains highly contentious as a biological model of brain-based consciousness.

The electromagnetic force holds atoms and molecules together and thus is a determinant of atomic and molecular structure including protein turnover (Meijer:2018). In the pioneering days of quantum mechanics, pilot-waves guided by the electromagnetic field waves were developed in the 1920s mainly through so-called Bohr-Kramers-Slater model. However, the pilot-wave theory of de Broglie-Bohm (see De:1987 and (Bohm:1990) for a review) is independent of the electromagnetic field. The pilot-wave theory represents a more fundamental theory whereupon an electron is a particle at its core and an active field surrounds the core where the latter guides the motion of the electron referred to as a guiding wave. This active field represents internal energy is due to ‘hidden’ thermodynamics according to de Broglie’s wave theory. (Bohm:1952) called this energy his ‘quantum potential’. The de Broglie-Bohm theory is applicable at the sub-atomic scale (10 {}^{-15} m) but may produce changes of negligible importance at the atomic scale of 0.1 nm (10 {}^{-10} m) or above, including the macro-quantum realm. This realm borders quantum physics and quantum chemistry. It can be quantified to be the scale where quantum processes prevail over classical processes.

The subtle changes inherent at the macro-quantum realm can be deciphered in terms of phase differences in thermo-quantum oscillations of electron clouds. Their existence depends on whether thermo-quantum fluctuations dominate over thermal fluctuations (Brownian motion). According to (Beck:1992) fewer than ten electrons suffice to ensure quantum processes prevail over thermal processes at typical length scales of the order of several nanometers. The classification of fluctuations as thermo-quantum or thermal depends on the thermal de Broglie wavelength expressed as follows:

\lambda =\sqrt{\frac{2\pi {\mathrm{\hslash }}^2}{\mathrm{m}{\kappa }_{\mathrm{B}}\mathrm{T}}}
where \mathrm{\hslash }=\frac{h}{2\pi } is the reduced Planck constant ( h=6.6\times {10}^{-34} Js is the Planck constant), m = 9.10 \times {10}^{-31} kg is the mass of electron multiplied by the number of electrons, {\kappa }_B=1.38\times {10}^{-23} JK {}^{-1} is the Boltzmann constant and T = 310 K is the body temperature. For example, if m = 10 electron masses and T = 310 K it follows that the thermal de Broglie wavelength is a few nanometers. Hence if the average molecular dipole-bound electron distance ( \mathrm{L} ) at scales of few nanometers \lambda \sim \mathrm{L} then the fluctuations can be treated classically. However, for greater number of electrons with an average molecular dipole-bound electron distance, for example at the femtometer scale \lambda \gg \mathrm{L} , thermo-quantum fluctuations dominate.

The de Broglie-Bohm theory being observer independent portrays consciousness to be not fundamental but arising from the intrinsic constituency of matter. In a non-reductionist perspective, consciousness co-exists with cognition and has an affect according to “biological naturalism” (Searle:2007). What this means is that consciousness has no causal attribute to effect cognition due to its lability, but consciousness affects the brain for cognition to decode consciousness in memory as conscious experience (self-awareness). The affective role stems from the composite of magnetic dipole moments forming an electromagnetic field as opposed to an effective role in the presence of a pre-existing electromagnetic field associated with cognition. Note that, free electrons are evanescent in the cerebral fluid. Excited water molecules are destroyed with the formation of ions, and electrons capture water molecules and become solvated: H {}_2 O ⟶ H {}^{+} + OH {}^{-} . The mass of the ion OH {}^{-} is about 16 masses of the hydronium ion H {}^{+} is \sim 2.7 ×10 \times {10}^{-26} kg. From Eq. 1 it follows that \lambda \sim 1Å. That is, this ion can migrate on a length comparable to the intermolecular spacing, about 7 Å. Having lost most of the energy for excitation and ionization of water molecules, the electron continues to interact with the surrounding water molecules until it is in the potential barrier, since it cannot overcome the electrostatic repulsion of the electron cloud of the molecule through which it passes. In this case, the free electron is in the region of relatively low potential energy, i.e., in the intermediate position corresponding to the element of free volume in the fluid where it is stabilized by short-range repulsive forces.

Where in neurons could such non-electrolytic regions exist? As shown in Fig. 1, proteinaceous structures have apolar (hydrophobic) regions, for example in an enzymatic cleft (Mentre:2012). These proteinaceous structures found in thin cylindrical nonsynaptic spines of cortical interneurons are isolated from neuronal (synaptic) variability, especially thin sized nonsynaptic spines, approximately 0.09 \mu m in diameter compared with the larger pyramidal neurons of the neocortex (Arellano:2007). Particularly, nonsynaptic spines with clearly absent synaptic-head like filopodia, containing cytoskeletal proteins bound to actin-filament (see Fig. 1). The nonpolar hydrophobic regions of proteins contain molecular dipole-bound electron densities that can undergo phase difference oscillations that differ from the polarized regions along actin filaments (Tuszynski:2004). Macro-quantum potential energy in nonsynaptic spines are theorized to carry intrinsic information through a nested hierarchy of nonconscious microprocesses. It is further suggested that ‘binding’ of intrinsic information would be en route for decoding in memory. The functional actin-filaments that are found in the cortical region near the membrane of neurons, while the actin-binding proteins that fill nonsynaptic spines were first proposed by (Woolf:2011) as a possible seat of biological consciousness.

Figure 1.

Schematic diagram illustrating (top left) a neuronal branchlet studded with spines and (top right) shows a nonsynaptic spine shaft containing cytoskeletal molecular proteins bound to actin-filament networks within the spine shaft of uniform length approximately 0.7 \mu m and 100 nm in diameter. The quantized subspace is where pilot-waves originate at picometer scale ( \sim 0.1 nm) within apolar hydrophobic regions of actin-binding proteins shown (bottom left) form clathrate-like structures with water molecules (adapted from Mentre:2012. The electron clouds at \sim 10 pico-meter scale (bottom right) is an order of magnitude smaller than most chemistry transformations and measurements and therefore below physical chemistry.

Quantum chemistry underpins the so-called ‘quantum underground’ where consciousness originates (see (Craddock:2017) for a review). Due to inherent uncertainty in electron localization, the weakest type of Van der Waals force, the so-called London force, exhibit quantum effects and the resultant dipole oscillations (see (Hameroff:2008) for a review). London forces are quantum level instantaneous, but weak, induced dipole-induced dipole couplings, due to charge separation resulting in attractive Coulomb forces occurring between nonpolar \pi -electron resonance clouds of two or more neutral atoms, molecules or macromolecules. Nonpolar hydrophobicity signifies absence of electrostatic forces and hydrogen bonding. In nucleic acids and less commonly in proteins, ‘benzene-rings’ are stacked precisely on top of each other and hence support short-range interactions through London forces. It is only ring-like structures like ‘benzene-rings’ that can support oscillatory motion back and forth due to shared electron pairs between two carbons, based on free electrons in \pi -electron resonance clouds. (Hameroff:1982) postulated that London force dipoles in nonpolar hydrophobic regions of cytoplasmic proteins couple and oscillate coherently, and this coupling was suggested to be necessary for consciousness.

(Hameroff:1983) hypothesized that anesthetics bind in nonpolar hydrophobic regions of cytoskeletal proteins, dispersing endogenous London forces by inhibiting free electrons in \pi -electron resonance clouds. (Franks:1984) found that anesthetics acts directly within cytoskeletal proteins that bind to actin-filaments in spines (see also Kaech:1999). Actin-filaments are found along the plasma membrane of neurons and profusely fill dendritic spines (see (Hotulainen:2010) for a review). As a result, macroscopic description of subneuronal dynamics that can provide a novel physical attribution to ‘long-range’ coherence in biological systems (Preto:2016) where synchrony has been invoked as a neural basis for emergent consciousness (Hameroff:1982, Hameroff:1994, Tory:2018) as well as a precedence for unitary binding of consciousness (Crick:1994a) resulting in a unified conscious field (Searle:2017).

However, London forces cause dipole oscillations, but not necessarily the phase-differences of the oscillating molecular dipole-bound electrons succinctly referred to as quasi-free electron density distribution in proteins bound to actin filaments. Accordingly, an alternative explanation which is below physical chemistry is required. When using de Broglie-Bohm approach, we escape the need for binding and quantum coherency in signaling at the atomic scale. The formation of a macro-quantum state is a way of preventing against dissipation and could be the result of pairing molecular dipole-bound electrons of opposite spins, enabling the formation of strong bonds for atoms to form molecular ions. Spin pairing in molecules is dependent on the Pauli exclusion principle, yet molecular dipole-bound electrons can aid the formation of a macro-quantum state through quantum (electron) tunneling by way of a chiral potential (or selective guidance of electrons by the accompanying magnetic field) selecting spin polarizations in a dynamic network of atoms (Naaman:2016, Michaeli:2016, Banerjee-Ghosh:2018). which may give rise to more ‘electron smears’ without the need for quantum coherence.

We also assume that guidance waves and their resultant thermo-quantum fluctuations govern subtle internal energy leading to hierarchical thermodynamic transfer of information in the realm of preconscious. What is transferred from the macro-quantum realm to the classical realm is information (theoretic) entropy as thermo-qubits containing information on the phase relations between molecular dipole-bound electron clouds of multiple systems. A pattern of macro-quantum potential energy entails the transfer of thermo-qubits in the macro-quantum realm where the process of thermo-quantum fluctuations is driven by de Broglie ‘hidden’ thermodynamics (De:1970). Thermo-quantum fluctuations occur at quantum equilibrium as phase-differences of dipole-bound electrons due to active fields of electron clouds, which guides their motion involved in producing thermo-qubits. The information we are discussing is intrinsic in the sense that it remains ‘hidden’ from the operational explanations of cognitive capacities. It is neither quantum information nor integrated information, but interconnected ‘intrinsic’ information associated with the internal thermo-quantum fluctuations that arise due to guidance waves transferring thermo-quantum internal energy by means of the macro-quantum potential Grr:2009, (Nottale:2014). This internal energy in the brain originates beneath the realm of physical chemistry and is influenced by the ‘shape’ of the enveloping field density of molecular dipole-bound electrons associated with de Broglie ‘hidden’ thermodynamics.

How are the microprocesses actualized to achieve qualia? Qualia represents the content of thermo-qubits and it is obviously a function of the nonconscious microprocesses, but there is no evidence for qualia in cognitive processing. If we perceive a blue bird, then the blueness is a quale. Yet, the essence of a quale is not the color tone through cognitive processing, but rather it is the actualization of the potential ‘feeling’. Therefore, qualia are the phenomenal ‘feel’ of cognition, yet never a function of cognition. The notion of ‘feelings’ constitute a multitude of discrete atomic microfeels synonymous with the philosopher Alfred Whitehead’s spatiotemporal chains of ‘occasions of experience.’ The experienceability process entails a continuum of patterns of discrete atomic microfeels (‘qualia’) that constitutes a continuous stream of intrinsic information in the preconscious. But to include qualities of a being, qualia must become part of cognition, which must constitute 'cognized content'. The connection between preconscious and its cognized content is through hierarchical thermodynamic transfer of information culminating in thermo-qubits, which is a specific energy²(2 Specific energy is energy per unit mass) transfer in the macro-quantum realm where thermo-quantum fluctuations operate through de Broglie ’hidden’ thermodynamics De:1987. Thermo-qubits exist for \lambda \gg \mathrm{L} and fade because of thermal agitation when \lambda \sim \mathrm{L} . They get transferred within a nested hierarchy of microprocesses at equilibrium and dynamical macroprocesses out of equilibrium (Kosloff:2013).

From the viewpoint of irreversible thermodynamics of the human brain (Kirkaldy:1965), the nested hierarchy of nonconscious microprocesses are ‘open’ dissipative systems, in the sense that there is elimination of entropy across hierarchical levels (i.e., quasi-thermodynamic processes out of equilibrium). Therefore, entropic exchange between hierarchical levels is assumed to take place by way of hierarchical thermodynamic transfer of information. Hierarchical thermodynamics includes weakly nonequilibrium thermodynamics, also known as ‘quasi-equilibrium’ thermodynamics for systems close to equilibrium. Although the second-law of thermodynamics states that entropy and internal energy can only be created, but not eliminated in an ‘isolated’ thermodynamic system, supporting local-equilibrium conditions (Collell:2015), hierarchical thermodynamics purports quasi-thermodynamic conditions in the sense that there is elimination of entropy across hierarchical levels (i.e., spontaneous irreversible processes out of equilibrium). In other words, any microprocess consists of an operation cycle where the first stage of the entropic exchange between hierarchical levels is ‘closed’; thus, the thermodynamic transfer operates as an ‘isolated’ thermodynamic system and during the second stage the entropic exchange between hierarchical levels is ‘quasi-closed’; thus, the thermodynamic transfer operates as an ‘open’ dissipative system far from equilibrium (Del Castillo:2011).

The fundamental mechanism providing the change from potentiality to actuality may in principle be very similar for all nonconscious microprocesses involving hierarchic thermodynamic transfer of information (Mahler:2015). The de Broglie's 'hidden' thermodynamics (De:1970) plays a role in thermodynamic transfer of information across hierarchical levels. For example, entropic exchange is assumed to take place in dissipative structures far from equilibrium by way of transfer of thermo-quantum information as thermodynamic entropy. We now know that in such a system negative entropy can result, and order can emerge from disorder. For a wildly disordered system, large-scale order can emerge and stabilize, and the more chaotic the disorder, the greater the stability of the ordered patterns that emerge (Song:2018).

In a recent study, (Poznanski:2018) had used negentropy principle of information to bridge the explanatory gap (Levine:1983). This principle links decreases in information (theoretic) entropy with increases in thermal entropy (Brillouin:1953, Brillouin:1962). Therefore, this is not just a quantitative descriptor of the causal relations between measured events (subjective), but has a physical reality in terms of thermal entropy, which is an objective property of the brain (Collell:2015). The model states that brain-based consciousness is not solely based on quantum effects (Baars:2012, Hameroff:2014), but instead is a concomitant of dynamic patterns reflecting information (theoretic) entropy that is encoded from the lability in the phase differences of thermo-quantum fluctuations as thermo-qubits. Thermo-qubits carry intrinsic information through negentropic action within neurons which can be a cause of hidden communication.

In scientific foundations of neurology, consciousness is labile (Walshe:1972), but in clinical neurology, consciousness is often defined to be ‘on’ or ‘off’ as for example, when the patient is either asleep or in a coma and this observed by fMRI through coma-causing lesions in functional connectivity networks (Fischer:2016). The same applies to psychological perspectives or higher order theory of consciousness (Rosenthal:1997). However, such attempts at understanding consciousness are not grounded in the consciousness process as part of a spontaneous process. Theoretical work in this direction has been initiated by (Marchetti:2018) in which he details how consciousness is a ‘unique way’ of processing information, yet without demonstrating what this ‘unique way’ could be physically. As well, (Hameroff:2010) who first explored the idea of a ‘conscious pilot’ embodied in the activity of subneuronal structures forming a holoscope (i.e., cognized “wholes”). A very recent paper by (Schiffer:2019) points a way forward from the traditional medical definition of consciousness and puts forward the hypothesis that preconscious experienceability becomes subjective upon interaction with intrinsic information, while a further encounter with cognition leads to awareness thereby becoming full conscious experience (or the conscious Self).

In view of the above, we postulate a new theory on how the brain encodes consciousness based on ‘negentropic entanglement’ of intrinsic information. Negentropic entanglement binds nonconscious entities to ‘potential’ conscious entities, temporally, thus acting as an integrator of thermo-qubits conveying ‘meaning’ expressed at the fundamental level, arising at the atomic scale of matter from de Broglie ‘hidden’ thermodynamics. The ‘meaning’ of negentropic entanglement is ascribed to the negentropic action or experiential flow of macro-quantum potential energy in a nested hierarchy of nonconscious microprocesses as thermo-qubits reflecting internal thermo-quantum fluctuations associated with the lability in the phase differences of the molecular dipole-bound electron oscillations.

Quantum chemistry governs the behavior of electrons that are bound to atomic nuclei resulting in stable inter-atomic bonds in the formation of molecules. The guidance wave theory is at the atomic scale yet independent of quantum chemistry. A wave function has characteristics of a macroscopic quantum state referred to as ‘quantum-like’ (Gould:1995). It is not the standard wave function of quantum mechanics, used to measure potentialities, but describing the passage of a potentiality to actuality based on the wave function’s averaged statistical characteristics. The wave function ( \psi ) is dimensionless and describes the instantaneous state of the enveloping ‘active’ field density of molecular dipole-bound electrons modeled by the macro-quantum wave equation:

\mathrm{i}\gamma \frac{\partial \psi }{\partial \tau }=-\frac{{\gamma }^2}{2\mathrm{m}}{\nabla }^2\psi +\mathrm{U}\mathrm{\Psi }+\epsilon \left|{\mathrm{\Psi }}^2\right|\mathrm{\Psi }
where \epsilon is a parameter that regulates the strength of the nonlinearity in units of [energy], m is the mass of electrons in units of [mass] and the action parameter ( \gamma ) carries dimensions of [energy][time]. This is known as the modified nonlinear Schrödinger equation or nonlinear macro-quantum wave equation. Like the so-called the Gross-Pitaevskii equation (Barenghi:2016) that includes interactions through the addition of an interaction potential term |{\psi }^2|\psi may give more insight on the individual electron cloud interactions. Eq.2 when ϵ =0 is simplified to the linear Schrödinger equation and can be solved by substituting the Madelung transformation: \psi =\sqrt{\rho (\mathrm{x},\mathrm{t})}{e}^{\frac{iS}{\gamma }} into (Eq.2), upon separating the imaginary and real parts. The real part describes how the ‘shape’ of the enveloping field density of molecular dipole-bound electrons is represented by the phase differences of the oscillating molecular dipole-bound electrons expressed in terms of the Hamilton-Jacobi equation (Gould:1995):

\frac{\partial s}{\partial t}+\frac{1}{2\mathrm{m}}{(\nabla S)}^2+\mathrm{U}+Q=0
where S=\frac{\mathrm{i}}{2}\gamma \ln \frac{{\psi }^{*}}{\psi } is the action function of the phase differences of oscillating molecular dipole-bound electrons in units of [energy][time], {\phi }^{*} is the complex conjugate of the wave function and \nabla is the gradient (in one-dimension \equiv \frac{\partial }{{\partial }_x} ). The constant of inverse proportionality ( \nu ) in the relation \gamma =\nu /\nabla S carries dimensions of [length] [energy] [time]. The constant of inverse proportionality ( \nu ) between the ‘action’ parameter ( \gamma ) in units of [energy][time] and the gradient of the action function ( \nabla S ) in units of [energy][time]/[length]:

\nabla S=i\gamma (\frac{1}{\sqrt{{\mathrm{\Psi }}^{*}}}\frac{\partial \sqrt{{\mathrm{\Psi }}^{*}}}{\partial x}-\frac{1}{\sqrt{\mathrm{\Psi }}}\frac{\partial \sqrt{\mathrm{\Psi }}}{\partial x})
The action function is under the action of two ‘potentials’: (i) the classical potential energy function U(x,t) in units of [energy] and (ii) the macro-quantum potential energy in units of [energy]:

Q(x,t)=-\frac{1}{2\mathrm{m}}{\gamma }^2\frac{{\nabla }^2\sqrt{\rho (x,t)}}{\sqrt{\rho (x,t)}}
where \rho (x,t) is the enveloping field density distribution (dimensionless) , x is the length scale and {\nabla }^2 is the Laplacian (in one-dimension \frac{{\partial }^2}{\partial {\chi }^2} ). The macro-quantum potential (Q) like the quantum potential (Bohm:1990) is an emergent potential that is not explicitly given in Eq.3 until the solution of Eq.2 is found via the Born rule, linking the amplitude of the wave function to the enveloping field density distribution: \rho (x,t)={\left|\psi \right|}^2 . The Born rule implies quantum equilibrium conditions. Note: The macro-quantum potential applies to a single electron density cloud and it is not a super macro-quantum potential which applies in the case of several electron density clouds and how they interact together. This is a simplification of the model since we ignore such interactions between electrons in density clouds and between multiple electron density clouds. If we introduce the simple case of a static classical potential energy with a unitary value U=1 and letting \epsilon = 0 then the solution of Eq.2 is readily found, viz.

\mathrm{\Psi }(x,t)={\mathrm{\Psi }}_0(x,t)e^{-\frac{i}{\gamma }t}
and enveloping field density distribution \rho (x,t)={\left|\psi \right|}^2 where {\psi }_0 (x,t) satisfies the macro-quantum reduced wave equation:

\mathrm{i}\gamma \frac{\partial {\psi }_0}{{\partial }_{\mathrm{t}}}=-\frac{{\gamma }^2}{2}{\nabla }^2{\mathrm{\Psi }}_0
Eq.7 ignores the effects of the classical potential energy U=0 and considers solely the quantum effects of the molecular dipole-bound electron densities.

The separation of variables method yields a solution of Eq.7 in terms of spatial region of length (average molecular dipole-bound electron distance) L, subject to Dirichlet boundary conditions (Pribram:1991):

{\psi }_o(x,t)=\left(\frac{1}{L}\right){\sum }_{n=1}^{\mathrm{\infty }}{c}_n{e}^{-\frac{2}{\gamma }i\left(\frac{{\gamma }^2}{L^2}{\pi }^2{n}^{2}t-\frac{\gamma }{L}\pi \mathrm{x}\right)}
where c_n are Fourier coefficients independent of time in units of [length]. In the ontological interpretation of quantum mechanics, a ‘pilot-wave’ guides the enveloping field density of molecular dipole-bound electrons whose ‘shape’ determines their phase differences of the thermo-quantum fluctuations in a way, so it also acts as an ‘information channel’ through a context-dependent energy redistribution (Hiley:2002, Sbitnev:2009, Dennis:2015). Moreover, Q conveys meaning as an information channel and as a quantum ‘corrector’ of the total energy. The kinetic energy is \frac{1}{2\mathrm{m}}{(\nabla S)}^2 and from Eq.3 the total energy is -\frac{\partial S}{\partial t} .

With Born’s rule and application of the chain and product rules, Eq.5 becomes:

Q(x,t)=-\frac{1}{4\mathrm{m}}{\gamma }^2[\frac{{\nabla }^2\rho }{\rho }-\frac{1}{2}\frac{{(\nabla \rho )}^2}{{\rho }^2}]
and together with \nabla \rho /\rho =\nabla (\ln \rho ) Eq.9 reduces to:

Q(x.t)=-\frac{{\gamma }^2}{8\mathrm{m}}[\nabla {(\ln \rho )}^2]-\frac{{\gamma }^2}{4\mathrm{m}}[{\nabla }^2(\ln \rho )]
Intrinsic information is not Shannonian, but Fisherian (in the sense of uncertainty), and observer-independent thus it is computable. The non-computability in the sense of Gödelian information (Cicurel:2015) is a kind of intrinsic representation of the brain within cognition, representing intrinsic information via uncertainty in the brain. In the context of thermodynamic effects, Q is proportional to Fisher information (theoretic) entropy (measure of the uncertainty of data in an ‘information channel’) and the negentropic force can be explicitly determined from the gradient of the local Boltzmann thermal entropy (cf., Tsekov:2012). The negentropic force is due to thermo-quantum internal energy (Q) as per de Broglie ‘hidden’ thermodynamics arising from the microscopic scale of matter. In view of the negentropy principle of information (Brillouin:1953, Brillouin:1962), the negentropic action reflects a ‘consciousness guidance’ because information (theoretic) entropy is subjective at the scale of measurement. Therefore, Q in the context of thermo-quantum fluctuations, produces negentropic action, which is not a mechanical action but simply the action of S where negentropic action can be channeled through the negentropic force.

The quantum force F_Q=-\nabla Q has been evaluated based on the de Broglie-Bohm theory:

F_Q=\frac{{\mathrm{{\rm Y}}}^2}{4\mathrm{m}{\rho }^2}[\rho {\nabla }^3\rho -\frac{1}{2}\nabla (\nabla \rho .\nabla \rho )-\nabla \rho ({\nabla }^2\rho -\frac{\nabla \rho .\nabla \rho }{\rho })]
The negentropic action representing experiential flow of specific energy that results in a negentropic force can also be explicitly represented by the gradient of the local Boltzmann thermal entropy \nabla S_Q (Tsekov:2012). Defining the Boltzmann thermal entropy (Sbitnev:2009) as a logarithmic function S_Q=-\frac{1}{2}\ln (\rho ) and Eq.10 becomes:

Q(\mathrm{x},\mathrm{t})=-\frac{{\gamma }^2}{2\mathrm{m}}[\nabla {\left(S_Q\right)}^2]+\frac{{\gamma }^2}{2\mathrm{m}}{\nabla }^2{S}_Q
Here the first-term on the RHS is viewed as the macro-quantum ‘corrector’ of the kinetic energy term and the second-term on the RHS influences the classical potential energy term U in Eq.2. The kinetic energy becomes \frac{1}{2\mathrm{m}}{(\nabla S)}^2-\frac{{\gamma }^2}{2\mathrm{m}}[\nabla {\left(S_Q\right)}^2] and the classical potential energy becomes U+\frac{{\gamma }^2}{2\mathrm{m}}{\nabla }^2{S}_Q . This implies that the kinetic energy of the oscillating molecular dipole-bound electrons contains a negentropic term. Note: {(\nabla S)}^2 has unit of ({[energy][time]/[length]}) {}^2 = [mass][energy]. A mean approximation of the negentropic force determined directly by Eq.12 through F_Q=-\nabla Q is responsible for the of phase differences of the oscillating molecular dipole-bound electrons (cf. Dennis:2015; Heifetz:2016).

The negentropic force can be explicitly represented by the gradient of the macro-quantum potential energy F_Q=-\nabla Q , where Q is expressed by Eq.12 which is shown in Fig. 2. The real component indicates changes to the average phase difference of the oscillating molecular dipole-bound electrons, while the imaginary component signifies the movement of the phase differences from their average values. The sense of a negentropic force specifies the direction (positive or negative) in which the force moves along the line of action, which in Fig. 2 is shown that the real component of F_Q is both spatially positive and negative in amplitude. A negative amplitude of F_Q indicates the direction is reversed to the mean approximation. This implies that zero amplitudes reflect coherence, while non-zero amplitudes, designate incoherency necessary for the negentropic action (or experiential flow of thermo-quantum internal energy that results in a negentropic force) to be encoded. The negentropic action representing experiential flow of specific energy that results in a negentropic force appears to be on-going without dissipating, defining what is meant by negentropic entanglement. The movement from the average values has certain regions where F_Q is zero. However, the average values are never zero which is an indication that F_Q is continuous supporting the concept of negentropic entanglement.

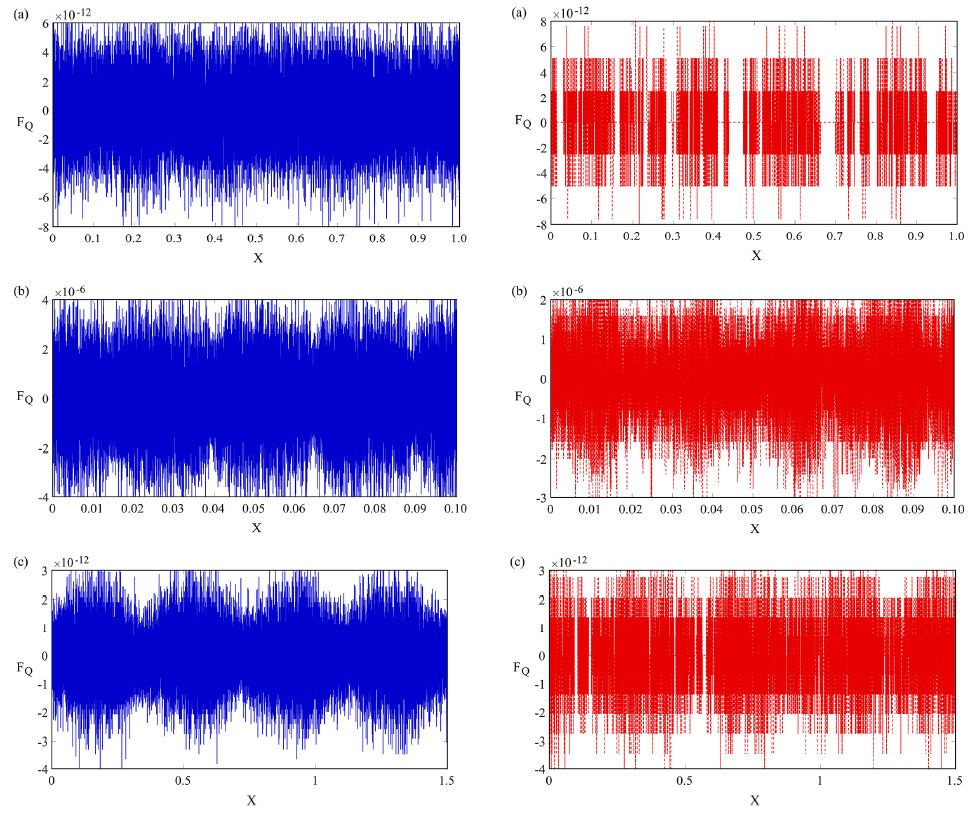Figure 2.

The mean approximation of the negentropic force in units of Newton per unit mass as a function of space resulting from the macro-quantum potential energy (Q) for (a) L = 1, t = 0.1, \gamma = 1.0 (b) L = 0.1, t = 0.01, \gamma = 0.01. and (c) L = 1.5, t = 0.5, \gamma = 2. Real component is shown in blue and imaginary component is shown in red. The values of the parameters were arbitrarily chosen.

A temporal variation in the mean approximation of the negentropic force is shown in Fig. 3. The nonzero-real values of F_Q indicate that mean approximation of the negentropic force can be used to encode changes to the average phase differences, while a non-zero imaginary component signifies the fluctuation from this mean-approximation of F_Q . The sense of continuity with time in both real and imaginary component of F_Q comes about from the phase differences of thermo-quantum fluctuations guided by the macro-quantum potential energy. The mean approximation of the negentropic force as given by the non-zero real value of F_Q signify the phase differences of the oscillating molecular dipole-bound electrons. They can differ from coupled molecular dipoles that oscillate between different orientations associated with endogenous London forces over long distances.

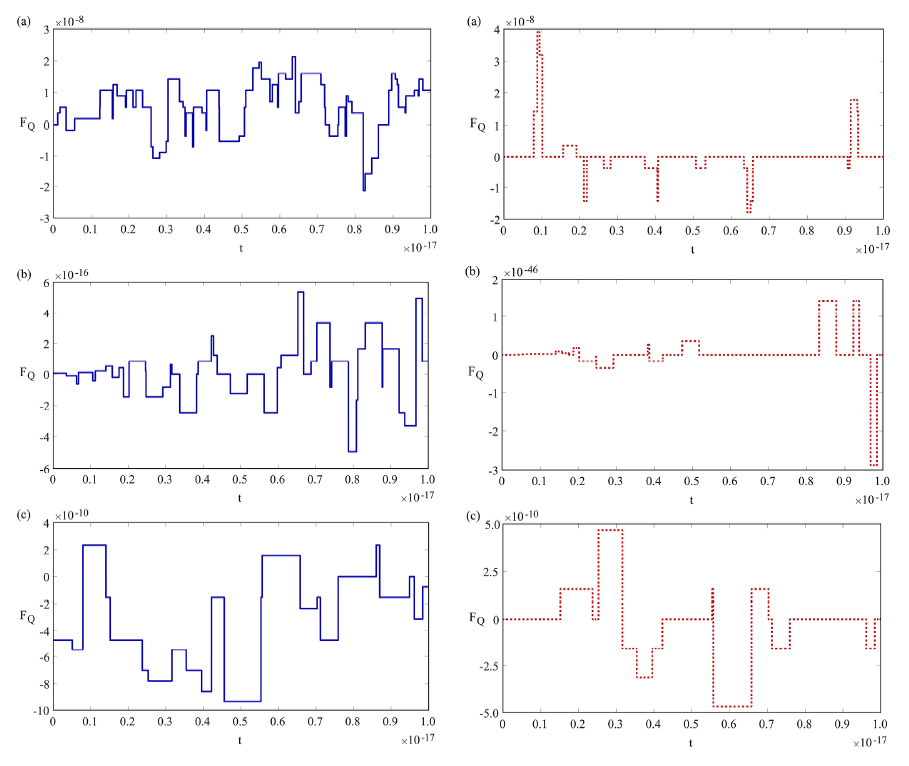Figure 3.

The sense of the negentropic force in units of Newton per unit mass as a function of time resulting from the macro-quantum potential energy (Q) for (a) L = 1, \gamma = 1.0, x = 0.1 (b) L = 0.1, \gamma = 0.01,x = 0.025 and (c) L = 1.5, \gamma = 2, x = 0.5. Real component is shown as a continuous line (blue) and imaginary component is shown as a dashed line (red). The values of the parameters were arbitrarily chosen.

The spatial variation in the mean approximation of the negentropic force at a smaller scale to Fig. 2 is shown in Fig.4. The amplitude of F_Q depends on the typical length scale (see Eq.1). The smaller the length scale the greater is the magnitude of negentropic force. When L = 0.1 (b) the amplitude is significantly greater compared when L = 1 (a) and L = 1.5 (c). We can surmise from this result that at smaller length scales the quantum processes prevail to reveal significant thermo-quantum fluctuations, while at larger length scales, less significant thermo-quantum fluctuations occur. The ‘consciousness code’ arises when quantum effects are not negligible and phase differences of thermo-quantum fluctuations of the oscillating molecular dipole-bound electrons are evident. When the thermal de Broglie wavelength ( \lambda ) is much smaller than the average molecular dipole-bound electron distance (L), the fluctuations can be treated classically as thermal fluctuations. On the other hand, when the thermal de Broglie wavelength ( \lambda ) is on the order of or larger than the average molecular dipole-bound electron distance (L), quantum effects will dominate, and the fluctuations must be treated non-classically as thermo-quantum fluctuations.

Figure 4.

The sense of the negentropic force in units of Newton per unit mass as a function of space resulting from the macro-quantum potential energy (Q) for (a) L = 1, \gamma = 1.0, t = 0.1 (b) L = 0.1, \gamma = 0.01, t = 0.01 and (c) L = 1.5, \gamma = 2.0, t = 0.5. Real component is shown as a continuous line (blue) and imaginary component is shown as a dashed line (red). The values of the parameters were arbitrarily chosen.

A relation between consciousness and negative entropy was proposed by (Song:2018) where the latter was declared as a ‘visible image of invisible consciousness’. The negentropic action can be channeled through the negentropy information principle as a ‘consciousness guidance’ consisting of dynamical patterns reflecting thermo-qubits. The ‘consciousness guidance’ is labile. Both real and imaginary components of F_Q have unique patterns that may signify informational content influenced by the ‘shape’ of the enveloping ‘active’ field density (cf. Figs. 3-4). This continuous real component of the mean approximation of the negentropic force points to continuous streams of information (theoretic) entropy (subjectivity) that are encoded in diverse way from the lability in the phase differences of thermo-quantum fluctuations. Due to application of the negentropy information principle, a ‘consciousness guidance’ arises from the gradient of the local Boltzmann thermal entropy ( \nabla S_Q ). The quantum ‘corrector’ of the kinetic energy -\frac{{\gamma }^2}{2\mathrm{m}}[\nabla {(S_Q)}^2] of the oscillating molecular dipole-bound electrons contributes to the ‘consciousness guidance’ since information (theoretic) entropy is subjective at the scale of measurement. The ‘consciousness guidance’ is encoded through the non-zero real component of the mean approximation of the negentropic force to form a ‘consciousness code’.

We clearly advocate that internal energy in the brain has a capacity for ‘feeling’ or experienceability without any claim that such preconscious episodes are a fundamental property of nature, providing the quality of that which is felt, i.e., a quale. This is against the ‘anthropic principle’ that claims consciousness is fundamental (cf. panpsychism). Panpsychist materialism portrays matter as inert as well as consciousness and therefore there is no other way to account for consciousness.

Our work based on panexperiential materialism considers matter not to be inert, but fluid-like, manifesting its influence at the atomic scale of matter through the action of guidance waves that originate from properties of electron clouds in brains. Thus, panexperiential physicalism is not mutually incompatible to materialist physicalism and hence what we call ‘panexperiential materialism’, which is causally efficacious in the preconscious. The noninertness of matter due to experiential flow of internal energy in the preconscious is associated with a nested hierarchy of nonconscious microprocesses, which is actualized as a continuum of patterns of discrete atomic ‘microfeels’(‘qualia’) (Holmgren:2014). Panexperientialist physicalism (Griffin:1997) considers actualized differences in energy to be the cause of consciousness. There is, however, a distinct difference between the notion of conscious experience and what panexperientialism claims to be preconscious experienceability.

The idea that if conscious processes are indeed physical processes, then there is something it is like, intrinsically, to undergo certain physical processes (Nagel:1974). (Pepperell:2018) argued for consciousness as a physical process caused by the organization of energy in the brain. Accordingly, it is specific energy transfer described in terms of actualized differences that supervene on neuronal activity. Although there is nothing in the brain that supervenes on the physical brain processes, nonconscious processes are physical brain processes too, but at a different hierarchical level in accordance with nonreductive physicalism the supervenience is ‘unpacked ’through hierarchical thermodynamic transfer of information.

Consciousness according to “biological naturalism” has no causal power (Searle:2007, Searle:2017). Therefore, transmutation of thermo-quantum fluctuations to normal-level neural signaling (MacGregor:2006) is inconsistent with quantum indeterminacy principle (Lewis:2006). As well, based on the indeterminacy principle, thermo-qubits cannot be amplified by electromagnetic fields interacting with cognition. This is because polarized regions associated with cognitive neural signaling are completely omniscient of guidance waves, whose abode is hypothesized to be in nonpolar hydrophobic regions of cytoskeletal proteins bound to actin filaments. Therefore, our theory is not a dual-aspect interactionist theory of mind in which the mind is an energetic source and the mind-brain interaction occurs through a binding of an electromagnetic field.

The higher phase of consciousness expressed through conscious perception and decoded in memory remains to be explored. The model supports the pioneering ideas of mathematical biologist Alfred Lotka’s conceptualization of consciousness not evolving from lower cell to higher cell organisms given an “elementary flash of consciousness may be a native of matter” (Lotka:1956), but rather it is the evolution of organisms that has allowed consciousness to be decoded in memory and therefore expressed. Preconscious experienceability is not expressed through perception like conscious perception must be. Thus, the notion of subjectivity comes from the intrinsic information and not from outside of the brain. This intrinsicness does not evolve, but is inherent in qubit-like properties of electron clouds. For this reason, we have advanced panexperiential materialism, where biological evolution is bounded by a materialistic pre-existence of the physical nature of subjective experience. That is, the popular notion of emergence of consciousness has no precedence in the solution of the mind-brain problem.

In this paper, we described how the internal energy processing is encoded to form a ‘consciousness code’ through the negentropy principle of information. In accordance with panexperiential materialism, the experiential flow of macro-quantum potential energy was realized through the nonzero real component to the mean approximation of the negentropic force. Such negentropic action was continuous in terms of its spatial distribution implying ‘binding’ of intrinsic information that we defined to be ‘negentropic entanglement’. By way of negentropic entanglement of hierarchical thermodynamic transfer of information as thermo-qubits, we have shown the essential process of experienceability in the preconscious.

We thank Alex Hankey, Jan Holmgren and Valeriy Sbitnev for detailed comments. Phichai Youplao for technical assistance. Funding support from UTM (FRGS-4F891) is greatly appreciated (J.A).

The authors declare that there is no conflict of interest regarding the publication of this article.